Jindřich Nový
Red Hat geek page 		avoid using my coding style:
int f,u=32,n[1024];s(v){if(u/=2)s(v),s(v|u),s
(v|u<<5);n[v]=u=u?u+u:1;};main(){for(f=~1,s(0
);f<1024;printf(n[++f]?f&31?"#":"\n#":" "));}
 home   personal   geo visualizations   volume rendering   quantum mechanics 

During my stay in Italy I was interested in solutions of non-relativistic time dependent Schroedinger wave equation
for a single particle. Since this equation is actually an equation of motion of a particle, the following animations show
how a partice (e.g. an electron) will interact with various types of potential.
(simplified - some more info will be [hopefully] added to bottom of the page).

The new animations show how it looks like in 2D:

Interaction with repulsive gaussian potential. Interaction with repulsive square potential.

Acceleration by an attractive gaussian potential. Probability density with expected particle position and phase.

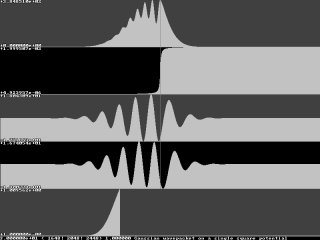
Tunneling of a particle beyond a potential barrier The same, but differently visualized.
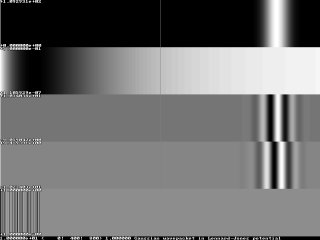
Particle "teleportation" to the minimum of potential The same, but differently visualized.

send beer and pizza to